In yesterday’s FT, “All in this together” assessed the possibility of a roughly synchronized downturn in the world’s major economies, with the United States, ironically enough, suffering the smallest hit. This brings up all sorts of interesting questions regarding exchange rates, if one believes that Taylor rules define monetary policy making to some degree, and that interest differentials affect exchange rates.
First, consider the evidence. The Euro area and Japan experienced negative growth in 2008Q2. The US experienced rapid GDP growth, but the consensus is for far more tepid growth in 2008Q3 (John Kitchen‘s estimate based on data available on 9/5 indicates 0.3% SAAR growth, although the underlying growth rate is 1.7%).
To the extent that monetary authorities respond to economic slack with lower interest rates, then one should anticipate lower interest rates in the Euro area and Japan as slow growth drives the output gaps negative in these regions (for a discussion of the distinction between growth and output gaps, see this post). However, it’s not clear to me that contemporary (i.e., in the past ten years) Japanese monetary policy is adequately modeled in such a way, so let’s take that off the table (but we can add in the UK).
In this post, I discussed how well a Taylor rule based model explained movements in the USD/EUR exchange rate. To the extent that this type of model works well for USD/GBP as well, what we should expect to see is a strengthening of the dollar as Euro area and UK interest rates fall. Here’s the 4 quarter ahead forecast from a slightly revised version of the model:
st-st-4 = 0.060 – 3.119 ogt-4 – 6.703 pit-4 – 0.020 (qt-4-0.109) – 6.546 ((qt-4-0.109)3) + 1.25 it-5 + ut
where og is the relative output gap, and pi is relative inflation, and q is the real exchange rate gap. The adj.R2 = 0.43, SER = 0.076, sample 1994q1-08q2; bold face indicates significance at the 10% level [update: using HAC robust standard errors].
If the reaction functions stay unchanged, then the implied 4 quarter ahead forecast is as depicted in Figure 1, i.e., a dollar appreciation over the next year ($1.42 by end-June 2009, vs. $1.58 end-June 2008).
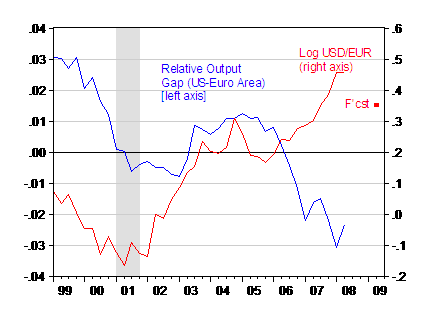
Figure 1: US-Euro Area output gap (blue, left axis), log dollar/euro rate (red, right axis), in-sample 4 quarter ahead prediction from Taylor rule fundamentals based on 2008Q2 data (red square, right axis). NBER defined recession dates shaded gray. Output gaps estimated as deviations from quadratics in time. Source: author’s calculations.
Here begins the speculative portion of this post (so no predictions here — just some musings.)
There is an interesting feature of the model, insofar as the target real exchange rate is included in the central bank reaction functions. As long as the target real exchange rate remains constant, the model should be stable; and indeed estimation of the model is predicated upon no-parameter-shifts. However, should the target shift (let’s say be depreciated from the US perspective), this will work to offset the Euro area and UK currency depreciation, and hence prevent expenditure switching toward those regions.
Now, would the target real exchange rate ever shift for the US? Followers of the Taylor rule literature know that shifts in targets are not typically contemplated. And indeed, it is debatable whether the Fed includes such a target in its Taylor rule.
That being said, we know that net exports accounted — in a mechanical sense — for almost all of the GDP growth in 2008Q2 [1]. About half of that is from import compression, and the other half is from export growth. To the extent that this is the only sector in the US sustaining growth, one could see the temptation to allow for a looser monetary policy. And as growth slackens in the rest-of-the-world(see Figure 2), surely the temptation will only strengthen.
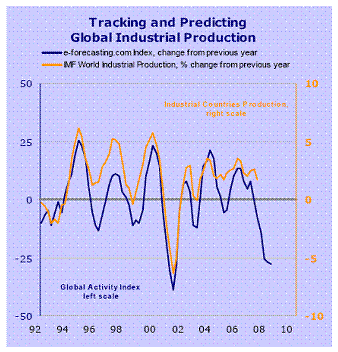
Figure 2: e-forecasting global activity index, and IMF industrial country index, Sept. 8, 2008.
Now consider what happens if the ECB or BoE also shift (depreciate) their target real exchange rates. That would mitigate the incipient dollar depreciation. In this case, interest rates are driven ever further downward — in the short run (with some lag, inflation will rise, stopping that effect).
This scenario is merely a modern version of the “competitive devaluation” phenomenon often used to interpet international monetary policies during the interwar period.
As I said before, no predictions here. Just something to think about. (As a parenthetical aside, if we end up extending EGTRRA and JGTRRA on top of adding in another $1.3 trillion in tax cuts, piled on top of whatever liability the Treasury will end up after the Fannie/Freddie bailout, then you can skip all the above text; you won’t need any monetary policy to drop the dollar’s value — all those structural budget deficits and associated debt will do the trick.)
[Update: 4:35pm, 9/11, posted in response to GNP’s query]
Yes, there is serial correlation, as indicated by the standard Q-statistic. I should have mentioned that the standard errors used to judge statistical significance are HAC robust standard errors. Below is the requested graph.
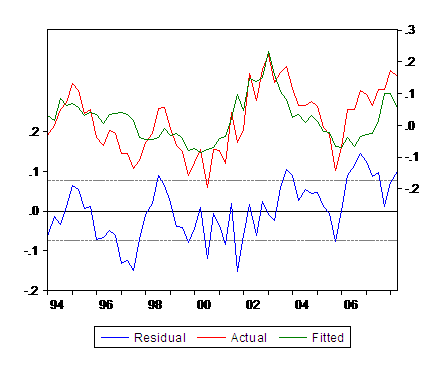
Figure 3: Actual (red), fitted (green) and residual (blue) from equation.
That being said, we know that net exports accounted — in a mechanical sense — for almost all of the GDP growth in 2008Q2
While certainly true for Q2 in some sense, I just wanted to explore this a bit.
Although GDP = C + I + G + E minus M,
Its also true that imports are not part of GDP.
Theyre only subtracted as a macro variable because they arent subtracted at the micro level in the preceding aggregation.
So a larger or smaller M, other things held strictly equal, doesnt change GDP.
Because imports are not part of GDP, is it really correct to posit functional relationships using imports as the argument, as we often do for GDP or GDP growth?
By extension, is it really correct to do the same thing for the quantity (E minus M)?
(E minus M) is obviously useful as a flow of funds variable but shouldnt it be used more carefully in GDP assessments?
E.g. a larger or smaller M, other things held strictly equal, will change the current account deficit, but as noted above, not GDP.
Did you mean anything like this when you used the words mechanical sense?
Isn’t the $XEU already at $1.42? With trendlines, I’ll go for $1.37.
Menzie,
Budget deficits and the value of the dollar and interest rates do not correlate very well. The late 1970s saw the debt to gdp ratio drop yet interest rates went up and the value of the dollar dropped. The opposite occurred in the 1980s; large deficits, falling interest rates and a strenghtening dollar. Even the last few years contradict conventional thinking. The public debt to gdp ratio was falling while the dollar was losing value. Now the budget deficit is predicted to increase to $400-500B and the dollar is improving. Based on history, I would not speculate, predict, or assume anything about the relationship between budget deficits and interest rates or the dollar.
Sorry,
Forgot to put my name (markg) on my post (anonymous at 06:31).
anon: I meant changes in net expors accounted for almost all GDP growth in exactly the sense you mentioned — in an accounting sense.
br: Yes, we’re at $1.41 today, but there’s no particular reason to be believe we’ll be there at end-June 2009. The correlations I’m exploting pertains to 4-quarter changes and the Taylor rule fundamentals. One might believe in “long swings” (slightly more sophisticated than trend lines), but I’m not sure a Markov-switching procedure would necessarily identify the move from $1.60 to $1.42 as a regime switch.
markg: Well, yes, a simple bivariate correlation would not verify a simple budget deficit/dollar story. But we don’t live in a bivariate world — and this is why we use multiple regression…
I do disagree with your characterization of publicly-held-debt. If you look at Figure 2 in this post, you will see that the trajectory of debt-to-GDP ratio changed drastically in FY2001, and is now approximately 5 ppts of GDP higher than it was in FY2001. And where is the dollar on a trade weighted basis relative to 2001? Looks about 35% in Figure 1 in this post.
“Budget deficits and the value of the dollar and interest rates do not correlate very well. The late 1970s saw the debt to gdp ratio drop yet interest rates went up and the value of the dollar dropped”
Whoever cooked up this concept was stupid:
Those who are wont to minimize the ill effects of the deficit are prone to compare the size of the deficit with nominal GDP, as if the volume of nominal GDP were independent of the size of the deficit. Unprecedented large deficits absorb a disproportionately large share of nominal GDP. Present deficits are unprecedented no matter how measured, and the past gives us no reliable guide to the future effects of deficit financing, beneficial or otherwise.
To appraise the effect of the federal budget deficit on interest rates, it is necessary to compare the deficit, not to the debt to GDP ratio (a contrived figure), but to the volume of current savings made available to the credit markets.
The 2006 deficit (406 billion interest expense) is absorbed about 23 percent of gross private domestic savings.
FY2006 total debt is the sum of: federal and state & local governments, international, and private debt, incl. households, business and financial sector debts, and federal debt to trust funds (50.5 trillion).
I think you are missing a very big piece of the picture when you go from the Taylor Rule to predicting exchange rate changes. Namely, the starting exchange rate matters. By the most fundamental standard (PPP) the eruo is too high. While a change in interest differentials can cause capital flows that dominate short-term currency moves, if the interest differential stays the same, once capital stocks have adjusted, the currency should migrate back to its fundamental value. So, I wouldn’t be at all surprised to see the euro at 120 within a year.
As for PPP, it is important to account for the VAT in Europe when comparing the euro to the dollar. This is a very big error in the Big Mac index that the Economist steadfastly refuses to acknowledge (beyond a vacuous list of caveats that includes taxes).
menzie: I know this is a quick ‘n dirty out-of-sample forecast and should not be taken too literally, perhaps more as a good basis for discussion and musings as you point out. But could you please post a graph of the residuals? Correct me if I’m wrong, but I’m assuming that the inferences and the forecast are based on the assumption of a normal or near-normal distribution.
P.S. I’m still chuckling at your previous Palin post and all the comments. Thank you!
don: You’ll note the level of the real exchange rate relative to target is in the specification.
Wait… No Iraq war stuff? Bush criticisms betwen the lines? Not even a Palin dig?!
Geez, now you’re not even trying’!!!!
Since on-topic comments have dried up, I would like to comment on the first commenters (anon) asssertion that, other things being equal, a large or small M (imports) does not impact GDP.
Impossible, if other things means the other variables in the equation defining GDP. If everything else remains the same on the right side of the equation except imports, GDP must change as imports change.
True, imports are not domestic production and they appear in the equation because consumption is not domestic production either. Imports reduce the size of consumption down to that part of domestic production, other than investment, consumed domestically.
This is important because domestic production is important. Imports force domestic production to be less than domestic consumption. If imports were less, and domestic consumption remained constant, domestic production would have to increase and GDP would be larger.
Of course, whether domestic production would fill the gap if imports decrease is an empirical question, but without the imports, we get either increased domestic production or less consumption. Either outcome would be better than sending more that 800 billion dollars overseas each year.
An on-topic comment. We are having a syncronized recession. A competitive depreciation is a possibility. The one certainity is that the U.S. is not going to be in a position to sustain a large trade deficit, as we have in the past 8 years, because the financial sector is no longer in a postion to produce the profits that sustained the growth in Net Wealth in the U.S. (despite billions of dollars going overseas) and attracted foreign investment to take advantage of the profit opportunities in the U.S. financial sector. The profits in the U.S. financial sector in the past decade (1997-2000 and 2003 – 2007) is my candidate for the force that first grew the value of the dollar through 2002 and then slowed its decline since 2002, in the face of the large twin deficits.
Those who think overseas investment in the U.S. is somehow paying for the U.S. trade deficit ignore the flow of assets other than cash that flow overseas in exchange for the cash sent to the U.S. to match our Current Account Deficit.
Re: don at 11:15 and your response at 11:29 –
Exactly. Don’t know how I missed it. Went back and revisited your earlier blog on the subject (in july), and found your model very properly predicted the sudden dollar rise we have seen, and in prophetic terms. (I was betting on it at the time, and was very glad to see the supporting argument.)
If you don’t bet on currencies, I hope you are able to profit from the good advice you have to offer others.
W. Raymond Mills:
“Impossible, if other things means the other variables in the equation defining GDP. If everything else remains the same on the right side of the equation except imports, GDP must change as imports change.”
Wrong. “Other things equal” means things other than imports, which is different than the other variables in the GDP equation.
Import content is embedded in the other variables. That’s why its subtracted. A change in what is subtracted is offset by an equal change in what is embedded, other things equal.
That’s why GDP doesn’t change, other things equal.
anon-
When consumption declines along with imports, GDP remains constant. But consumption decline is only one possibility. A more likely possibility is consumption constant, or nearly so, which would require an increase in domestic production and a consequent increaser in GDP.
At issue is not the meaning of “other things equal” which can be clarified by whatever definition you want to adopt. The real issue is the impact of import reduction on GDP, which will vary with the response of domestic producers and domestic consumers to the new reality.
Either Consumption is reduced or GDP is increased but we cannot simultaneously reduce imports while keeping constant consumption and GDP.
W. Raymond Mills,
I agree with everything you said.
Except that I defined the issue in this case to be other things equal relative to a change in imports, consistent with the clarification I requested in my first comment.
The point there being that the contribution of net exports to GDP is an accepted accounting device, but somewhat artificial in real economic terms. If a change in imports is an element in a net export change, it is also a potential element in all the other factors of the GDP equation. So the contribution of a net export change by definition has knock-on consequences beyond net exports. This is definitional, having nothing to do with secondary economic effects.
Further to my 11:41
E.g.
If net exports improve at the margin by one less item imported by Walmart, that alone has no effect on GDP, yet net exports are deemed to have made that contribution to any GDP change. (The import content of C is reduced by the same amount as the increase in (E – M)).
As per my point above, this ignores any associated true GDP change due to Walmart’s value added calculus, but I’ve left this aside as a separate issue in the other things equal approach.
(E – M) has integrity as a flow of funds component (current account contribution), but it really doesn’t as a GDP component.
You agree with everything I said. I said that import change will impact GDP change, depending upon what happens to consumption. If imports impact GDP change, that implies that imports have integrity as a component of GDP.
I was happy to leave the exchange until you added the unacceptable addendum.
“If imports impact GDP change, that implies that imports have integrity as a component of GDP.”
Absolutely not. Imports may or may not “impact” GDP change, depending on the response of domestic production to an import change.
But in any event, the fact that GDP can respond to an import change in no way implies imports have integrity as a component of GDP.
It’s entirely your choice to leave the exchange, happy or not.
Menzie wrote:
To the extent that this is the only sector in the US sustaining growth, one could see the temptation to allow for a looser monetary policy.
Menzie, do you seriously believe that the FED would lower rates from where they are?
anon;
If some larger or important point is at issue, the exchange perhaps should be prolonged.
I think the variables used to calculate GDP are each causal variables. If they were not, the calcuation of GDP would be erroneous, sooner or later.
If imports and exports are causal variables, the common argument that changes in the financial sector (financial flows formerly called The Capital Account) control or determine the size of the trade balance is wrong. I say Ben Bernanke was wrong in his argument that the U.S. trade deficit is due to a global savings glut. Instead, the excess global liquidity, in existence in 2005,was produced, in part, by the large U.S. trade deficit which sent cash overseas to pay for the excess of immports over exports.
Yes, this argument is worth pursuing, given that U.S. trade policy can be underminded if my postion is correct.
Please forgive my clumsyness. I am the author of the previous post. I just forgot to insert the correct information.
GNP: Good point; I’ve added some description and the requested graph at the end of the original post.
Anonymous/W. Raymond Mills: I did say that part of the post was completely conjecture. If the Fed does not include the real exchange rate in its objective function, then it’s meaningless to consider a shift in the target exchange rate.
On the other hand, what is true is that the current policy rate level is lower than that predicted by most estimates of Taylor rule functions for the Fed. So who knows.
W. Raymond Mills,
I agree with you on the causality of the current account balance and capital flows – surprisingly, because we agree, and because it is very much a minority view. I can’t possibly understand the argument of those who believe (virtually everybody) that capital flows cause current account imbalances, rather than vice versa. And I also reject the Bernanke savings glut thesis. But that is a separate issue from my perspective.
And I agree with you that imports can affect GDP.
My disagreement is that this functional economic relationship cannot be explained by the GDP accounting equation:
GDP = C + I + G + E – M
This equation can’t possibly explain GDP as a function of either net exports or imports. This is because import content is already included in the first four items, so that the net import content of GDP and this equation is 0.
So it is logically incorrect to assert that (E –M) contributes a certain amount to GDP growth, or that (- M) contributes a certain amount, on the basis of this equation. The presence of M in this equation is at best an accounting device, without much economic meaning.
This still allows for a functional economic relationship of the general form GDP = f (M, …), where f is some function that takes into account the effect of import related changes on domestic production responses.
But that functional relationship, which I think is your point, has nothing to do with the GDP accounting equation above, which is my point.
Bottom line I think is that we agree much more than we disagree, and the point of disagreement is fairly subtle.
W. Raymond Mills,
Here is a simplified example of my point.
Consider an economy where I, G, and E = 0.
So GDP = C minus M
And M = net exports
Suppose GDP changes from 100 to 101, and M changes from 10 to 9.
It would appear net exports M accounts for the entire growth of 1.
C remains unchanged at 110.
But C = C* + M, where C* is the domestically produced content of C.
So C* changes from 100 to 101.
So C* accounts for the entire growth of 1.
This is a contradiction.
Both M and C* can’t each account for the entire growth.
In fact, GDP = C minus M = (C* + M) minus M = C*.
GDP = C*, with 0 import content.
So C* accounts for the entire growth of 1.
M accounts for none of it – because M is not part of GDP or C*.
This is not to say that C* and GDP hasn’t been affected economically by the change in M, which is in agreement with your point.
Professor Chinn,
I would be interested to know if you think my last comment 5:00 a.m. is analytically correct or if I’m off base somewhere, because I can’t see where I would be wrong. Thx.
5:00 a.m. comment
net exports = minus M, of course
anon (5:06): The problem is that the expression:
Y ≡ C + I + G + E – M
is an identity, true by definition. Now take the total differential.
ΔY ≡ Δ C + Δ I + Δ G + Δ E – Δ M
The problem is that you have jerry rigged the problem so that changes in imports must manifest itself in a change in consumption. I can write the same expression you write:
C ≡ C*+M
But also impose a requirement that C* moves exactly in the same manner as imports. That would result in no seeming internal contradiction.
Now you’ll ask, why should consumption behave this way? That is exactly the point — accounting gives no guidance on what functional forms are appropriate.
That’s why it is important to distinguish between accounting and a formal model, and why formal models (by which I mean no more than a couple lines of functions and equilibrium conditions) are useful in thinking about what is going on.
I continue to be interested in this discussion but I would like to continue it between the two of us, if that is possible.
Will this blog permit me to post my E-Mail address? It is wrmills@wideopenswest.com. If you would like to continue, send me your E-Mail address.
Professor Chinn adds a useful perspective to our previous discussion. All agree that an increase in imports can have different impacts upon GDP, depending upon what impacts imports have on the other variables in the formula. Thus formal models are necessary.
One assetion of causal interdependence that needs further discussion is the notion that no formal model can be constructed which shows how an increase in imports can produce an increase in GDP. The whole issue of unbalanced traded needs further discussion.
W. Raymond Mills: I think you need a micro model wherein the benefits of comparative advantage, or increasing returns to scale in a differentiated product market, show up in order to get the results you posit. There are many of those sorts of models in the trade/growth literature.
Prof. Chinn
Thanks for responding. The total differential expression plus the distinction between accounting and a formal model are helpful devices.
W. Raymond Mills
Sorry, I didn’t mean to circumvent or pre-empt your input by asking for Prof. Chinn’s opinion. I much appreciate your helpful feedback and leaving your email address, but I think I’ll leave the discussion open here for the time being, for termination or not as you wish.
(The idea of a formal model showing the response of GDP to imports seems fairly intuitive to me, so I have no problem with that aspect, which must be one of the more interesting and essential in the analysis of trade and GDP.)
Both
You can probably tell I’m not an economist by training, but:
If I write:
GDP = C* + I + G + E
(Where, as earlier, C* = C – M)
And then if I think of GDP as a function of M, the distinction between the accounting model and the formal model becomes clearer to me.
By extension each component of GDP becomes a function of M.
So E is a function of M.
But GDP sensitivity in this case and normally it seems is being measured partly by the contribution of E minus M.
This still seems odd, in that it seems like GDP is being viewed as a function of E minus M in both an accounting sense and a formal sense, while E or E minus M in turn is a function of M in a formal sense.
Sigh … more to think about.
In the above 11:49, I should have said:
GDP = C* + I* + G* + E*,
where each component has been stripped of its respective M content
Professor Chinn – One must believe in the usefullness of the concepts of comparative advantage and increasing returns to scale to use those concepts in a model. In addition, my mind is attracted to simplier concepts.
Without equal trade, comparative advantage is a fiction. Comparative advantage assumes that resources displaced by import are able to find alternative employment. Unless that alternative employment (of financial as well as personnel) exists and can produce product that can sell on the internationl market, the trade deficit country suffers from excess imports.
I must rely on simplier models. I assume that all the variables on the right side of the equation that measures GDP are exogenous and causal factors in controlling the size of GDP. This is a model because it contains assumptions that allow for further deductions.
To me, the formula for GDP is the key to understanding what is going on in international trade.
Professor Chinn – I thank you for permitting me to get on at least one record a few of the basis assumptions I have develop while struggling alone for the last 5 years to understand international trade.
The causal assumption stated above is an oversimplification. Consumption can be an exogenous variable in some situations but part of it can be endogenous, depending upon how the domestic economy reacts to excessive imports.
Assuming the other variables are pure exogenous in the formula for GDP. the expression Savings equal Investment plus Exports minus Imports is interpreted as the causal factors on the right side of the equation. Savings is not a controlling variable in this equation. It is an edogenous variable – controlled by the other variables in the equation.
The article above seems to suggest that the US economy and the US dollar are doing better than the comparative economies oversees. The reason seemed to be lower interest rates by the Federal Reserve defined by the Taylor rule. It is important to understand that this is just speculation and the author provides no real argument other than the exchange rate of the US dollar. I will not go into this further because I think it is mute to argue that high volatility in exchange rates can reflect anything close to economic reality.
We are all in the process of grasping what kind of damage models can inflict on Wall Street and Main Street. Yet, we keep defending another model, the Taylor rule, which is just that, a model, and not what some might think an economic law. What’s more it is a deeply flawed model. There is no direct relation between output and employment on the one hand and the price of money on the other. Investment is an important factor of output but it is one of several as many astute readers have pointed out in their comments.
To re-inflate and lower the price of money is a short term patch at best and eventually leads to disaster, which will strike once authorities capitulate and are overwhelmed by the challenges they face. The current state of affairs perfectly fits this description. I am convinced that one of the changes that the crises that began in August of 2007 will bring about will be a changed Federal Reserve where the Taylor rule will not be used to determine interest rates in the future.
Note that Marc Farber has been wondering about this as well.